Answer:
1/2
Explanation:
We have
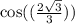 .
.
Let
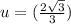 .
.
This implies
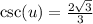 .
.
Use that sine and cosecant are reciprocals.
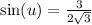
Now I'm going to rationalize the denominator there by multiply numerator and denominator by
 :
:

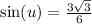
Reduce the fraction:
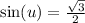
Now I'm going to use a Pythagorean Identity:
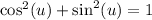 .
.
This will give me the value of cos(u) which would give me the answer to my question if it exists.
Replace
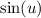 with
with
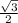 in:
in:
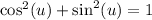

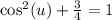
Subtract 3/4 on both sides:
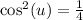
Square root both sides:
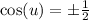 (since 1/2*1/2=1/4 or -1/2*-1/2=1/4)
(since 1/2*1/2=1/4 or -1/2*-1/2=1/4)
Now we must decide between the positive or the negative.
It depends where u lies. What quadrant? Hopefully it lays between 0 and
 . Otherwise, it doesn't exist (unless you have a different definition for arc function).
. Otherwise, it doesn't exist (unless you have a different definition for arc function).
So u led to this equation earlier:
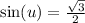
arcsin( ) only has outputs between
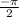 and
and
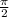 .
.
This would have to be in the first quadrant because we have only positive sine values there.
So this means cos(u)=1/2 and not -1/2 because we are using that u is in the 1st quadrant.
Remember u was
 .
.
So we have actually evaluated
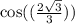 without a calculator.
without a calculator.
The value is 1/2.