Answer:
The point on the rim
Step-by-step explanation:
All the points on the disk travels at the same angular speed
 , since they cover the same angular displacement in the same time. Instead, the tangential speed of a point on the disk is given by
, since they cover the same angular displacement in the same time. Instead, the tangential speed of a point on the disk is given by
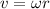
where
 is the angular speed
is the angular speed
r is the distance of the point from the centre of the disk
As we can see, the tangential speed is directly proportional to the distance from the centre: so the point on the rim, having a larger r than the point halway between the rim and the axis, will have a larger tangential speed, and therefore will travel a greater distance in a given time.