Answer:
n = 601
Explanation:
Since we know nothing about the percentage of computers with new operating system, we assume than 50% of the computers have new operating system.
So, p = 50% = 0.5
q = 1 - p = 1 - 0.5 = 0.5
Margin of error = E = 4 percentage points = 0.04
Confidence Level = 95%
z value associated with this confidence level = z = 1.96
We need to find the minimum sample size i.e. n
The formula for margin of error for the population proportion is:
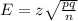
Re-arranging the equation for n, and using the values we get:
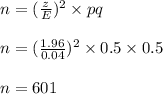
Thus, the minimum number of computers that must be surveyed is 601