Answer:
D.
![\log_4(3\sqrt[3]{35})](https://img.qammunity.org/2020/formulas/mathematics/middle-school/da1lhlf4wbhdfvpf7y8fdx88xy53q8upjm.png)
Explanation:
The given logarithmic expression is:
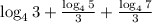
Don't let the fractions scare you at all.
We can rewrite the expression in another form that makes the fractions a bit friendly.
Recall that:
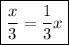
We apply this knowledge to get:
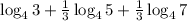
We can now use the following property:

We apply this property to get:
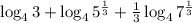
Recall again that:
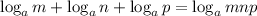
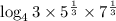
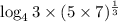
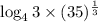
![\log_43\sqrt[3]{35}](https://img.qammunity.org/2020/formulas/mathematics/middle-school/j2t77fhqz1d4huyp7omxfwg8gx7tzl73xy.png)
The correct choice is D.