Determine whether QRS is a right triangle for the given vertices. Explain. Q(7, –10), R(–3, 0), S(9, –8) no; QR = , QS = , RS = ; RS2 + QS2 RQ2 yes; QR = , QS = , RS = ; QR2 + Q…

Question

asked Feb 5, 2020 206k views

1 Answer

Tom Norton · Answer 1 · 2020-02-08T15:22:48+0000

Answer:

yes; QR = √200, QS = √8 , RS = √208 ; QR^2 + QS^2 = RS^2

Explanation:

To determine the triangle as a right triangle we have to find the lengths of the sides

The distance formula will be used:

$d = \sqrt{(x_2-x_1)^(2)+(y_2-y_1)^(2) }\\ So,\\QR=\sqrt{(-3-7)^(2)+(0+10)^(2)}\\=\sqrt{(-10)^(2)+(10)^(2)}\\=√(100+100)\\=√(200)$

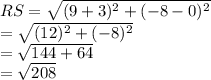
$RS=\sqrt{(9+3)^(2)+(-8-0)^(2)}\\=\sqrt{(12)^(2)+(-8)^(2)}\\=√(144+64)\\=√(208)$

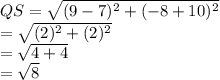
$QS=\sqrt{(9-7)^(2)+(-8+10)^(2)}\\=\sqrt{(2)^(2)+(2)^(2)}\\=√(4+4)\\=√(8)$

Using Pythagoras theorem, we can see that

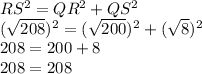
$RS^2 = QR^2+QS^2\\(√(208))^2=(√(200))^2 +(√(8))^2\\208 = 200+8\\208=208$

Which proves that given triangle is a right triangle ..