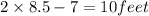Answer:
The width of rectangular floor shed =8.5 feet
Length of rectangular floor shed=
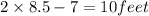
Explanation:
We are given that a floor is in rectangular shape.
We have to find the length and width of rectangular floor shed.
The area of floor shed=85 square feet
Let width of floor shed=x feet
Length of floor shed=(2 x-7 ) feet
Area of rectangle=
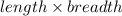
According to question
Area of rectangular floor shed=
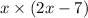
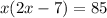
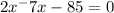
It is a quadratic equation
Using factorization method
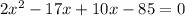
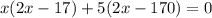


x=-5 is not possible because length and breadth of rectangle are always a natural number .
Therefore, the width of rectangular floor shed =8.5 feet
Length of rectangular floor shed=