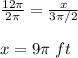Answer:
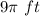
Explanation:
step 1
Find the circumference of the circle
The circumference is equal to
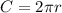
we have
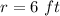
substitute
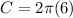
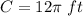
step 2
we know that
The circumference of a circle subtends a central angle of 2π radians
so
using proportion
Find out the length of the arc for a central angle of 3π/2 radians
Let
x------> the length of the arc