Question

32. Quality Control A campus bookstore buys 100 calcula-

tors. Assume that 2 have a defect of some kind. The math-
ematics department buys 8 of these calculators from the
bookstore. What is the probability that, of those calcula-
tors bought by the mathematics department,
(a) All are free of defects?
(b) Exactly 1 will have a defect?
(c) Exactly 2 will have a defect?

Answer 1

a) 0.8508

b) 0.1389

c) 0.0099

Explanation:

Total number of calculators bought = 100

Number of calculators with defect = 2

Probability of selecting a calculator with defect = p = 2 out of 100 =
`(2)/(100)=0.02`

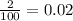
Probability of selecting a calculator without defect = q = 1 - p = 1 - 0.02 = 0.98

Part a)

The bookstore buys 8 calculators. This means the number of trials is fixed ( n =8). The calculator is either with defect or without defect. This means there are 2 outcomes only. The outcome of one selection is independent of other selections. This means all the events(selections) are independent of each other.

Hence, all the conditions of a Binomial Experiment are being satisfied. So we will use Binomial Probability to solve this problem.

We need to find the probability that all calculators are free of defects. i.e. number of success is 0 i.e. P( x = 0 )

The formula of Binomial Probability is:

`P(x) =^(n)C_(x) (p)^(x) q^(n-x)`

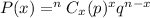
Using the values, we get:

`P(0)=^(8)C_(0)(0.02)^(0)(0.98)^(8-0)\\\\ P(0)=0.8508`

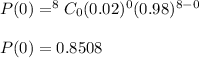
Thus, the probability that all calculators are free of defects is 0.8508

Part b) Exactly 1 calculator will have defect

This means the number of success is 1. So, we need to calculate ( x = 1)

Using the formula of binomial probability again and using x = 1, we get:

`P(1)=^(8)C_(1)(0.02)^(1)(0.98)^(8-1)\\\\ P(1)=0.1389`

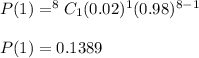
Thus, the probability that exactly two calculators will be having a defect is 0.1389

Part c) Exactly 2 calculator will have defect

This means the number of success is 2. So, we need to calculate ( x = 2 )

Using the formula of binomial probability again and using x = 2, we get:

`P(2)=^(8)C_(2)(0.02)^(2)(0.98)^(8-2)\\\\ P(2)=0.0099`

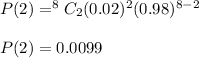
Thus, the probability that exactly two calculators will be having a defect is 0.0099