Question

X^4 - 1 =

A. (x+1)(x-1)(x^2+1)
B. ( X+1)^2(x-1)^2
C. (X+1)^3(X-1)^1
D. (x-1)^4

Answer 1

A. (x + 1)(x - 1)(x^2 + 1).

Explanation:

Using the difference of 2 squares (a^2 - b^2 = (a + b)(a - b) :

x^4 - 1 = (x^2 - 1)(x^2 + 1).

Now repeating the difference of 2 squares on x^2 - 1:

(x^2 - 1)(x^2 + 1 = (x + 1)(x - 1)(x^2 + 1).

Answer 2

A

Explanation:

Given

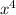`x^(4)` - 1 ← a difference of squares which factors in general as

a² - b² = (a - b)(a + b)

here
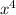`x^(4)` = (x²)² ⇒ a = x² and b = 1

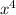`x^(4)` - 1 = (x² - 1)(x² + 1)

x² - 1 ← is a difference of squares and factors as

x² - 1 = (x - 1)(x + 1), so

(x² - 1)(x² + 1) = (x - 1)(x + 1)(x² + 1), hence

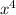`x^(4)` - 1 = (x - 1)(x + 1)(x² + 1) → A