Question

A cube has a net with area 24 in squared. How long is an edge of the​ cube?

Answer 1

6 is the correct answer

Answer 2

The length of the edge of the cube whose net area is 24 in sq is calculated as: 2 inches.

What is the length of a cube?

The net of a cube consists of six identical square faces. Let's denote the length of one side of the square as s.

The total surface area of the cube is the sum of the areas of its six faces. Since each face has an area of s², the total surface area (A) is given by:

`\[ A = 6s^2 \]`

You mentioned that the net has an area of 24 square inches. Therefore, we can set up the equation:

6s² = 24

Now, solve for s:

`s^2 = (24)/(6)`

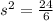
s² = 4

Take the square root of both sides:

`s = √(4)`

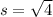
s = 2

So, the length of each edge of the cube is 2 inches.