Question

Can someone help me on this please:(?? I’m super bad at math!

Answer 1

Graph the two points (0,1) and (2,-1) then connect them with a straight edge.

Explanation:

The transformed graph is still a line since the parent is a line.

`g(x)=(-1)/(2)f(x+2)`

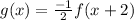
Identify two points that cross nicely on your curve for f:

(2,-2) and (4,2)

So I'm going to replace x in x+2 so that x+2 is 2 and then do it also for when x+2 is 4.

x+2=2 when x=0 since 0+2=2.

x+2=4 when x=2 since 2+2=4.

So plugging in x=0:

`g(x)=(-1)/(2)f(x+2)`

`g(0)=(-1)/(2)f(0+2)`

`g(0)=(-1)/(2)f(2)`

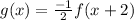
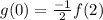
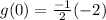`g(0)=(-1)/(2)(-2)` since we had the point (2,-2) on line f.

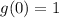`g(0)=1` so g contains the point (0,1).

So plugging in the other value we had for x, x=2:

`g(x)=(-1)/(2)f(x+2)`

`g(2)=(-1)/(2)f(2+2)`

`g(2)=(-1)/(2)f(4)`

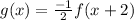
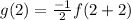
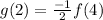
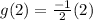`g(2)=(-1)/(2)(2)` since we had the point (4,2) on the line f.

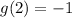`g(2)=-1` so g contains the point (2,-1).

Graph the two points (0,1) and (2,-1) then connect them with a straight edge.