Answer:
a. 435.4
Explanation:
We are given a geometric sequence with first term, firth term and the common ratio. We are asked to find the sum of first 5 terms of the geometric series.
The formula to calculate the sum of finite geometric series is:
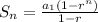
Since we need to find the sum of first 5 terms, n will be 5. Using these values in the above formula, we get:
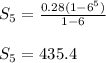
Therefore, the sum of first 5 terms of the given geometric series would be 435.4