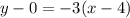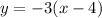What is the equation in point-slope form of the line passing through (4, 0) and (2, 6)? (5 points) y = 4x − 2 y = 2x − 4 y = −3(x − 4) y = 3(x + 4)

Question

asked Aug 17, 2020 159k views

2 Answers

Preben · Answer 1 · 2020-08-17T20:29:49+0000

Answer:

Third option:
y=-3(x-4)

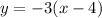
Explanation:

The equation of the line in Point-Slope form is:

y-y_1=m(x-x_1)

Where "m" is the slope and
(x_1,y_1) is a point on the line.

Given the points (4, 0) and (2, 6), we can find the slope with this formula:

m=(y_2-y_1)/(x_2-x_1)

Substituting values, we get:

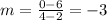
m=(0-6)/(4-2)=-3

Finally, substituting the slope and the point (4,0) into
y-y_1=m(x-x_1) , we get:

y-0=-3(x-4)

y=-3(x-4)

Patrick Goode · Answer 2 · 2020-08-24T04:45:58+0000

For this case we have that by definition, the point-slope equation of a line is given by:

$y-y_ {0} = m (x-x_ {0})$

We have the following points:

$(x_ {1}, y_ {1}) :( 2,6)\\(x_ {2}, y_ {2}) :( 4,0)\\m = \frac {y_ {2} -y_ {1}} {x_ {2} -x_ {1}} = \frac {0-6} {4-2} = \frac {-6} {2} = -3$

We chose a point:

$(x_ {0}, y_ {0}) :( 4,0)$

Substituting in the equation we have:

$y-0 = -3 (x-4)\\y = -3 (x-4)$

Finally, the equation is:
y = -3 (x-4)

Answer:

OPTION C