Question

Sqrt(x+4)=2-x
help me pleaseeeeeee

Answer 1

x = 0

Explanation:

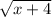`√(x+4)` = 2 - x ( square both sides to clear the radical )

x + 4 = (2 - x)² ← expand using FOIL

x + 4 = 4 - 4x + x² ( subtract x + 4 from both sides )

0 = x² - 5x ← factor out x from each term

0 = x(x - 5)

equate each factor to zero and solve for x

x = 0

x - 5 = 0 ⇒ x = 5

As a check

substitute these values into the equation and if both sides are equal then they are the solutions

x = 0

left side =
`√(0+4)` =
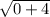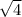`√(4)` = 2

right side = 2 - 0 = 2

then x = 0 is a solution

x = 5

left side =
`√(5+4)` =
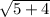`√(9)` = 3

right side = 2 - 5 = - 3 ≠ 3

then x = 5 is an extraneous solution

Answer 2

Answer:
`x=\Large\boxed{0}`

Explanation:

Given expression

`√(x+4) =2-x`

Square both sides of the equation

`√(x+4)^2 =(2-x)^2`

`x+4=4-4x+x^2`

Subtract x on both sides

`x+4-x=4-4x+x^2-x`

`4=x^2-5x+4`

Subtract 4 on both sides

`4-4=x^2-5x+4-4`

`0=x^2-5x`

Factorize the quadratic expression

`0=x(x-5)`

`x=\Large\boxed{0} \text{ or }x=5~(reject)\text{ }`

Check the answer

`\text{When x = 0:}`

`√((0)+4) =2-(0)`

`√(4)=2`

`2=2`
`\boxed{TRUE}`

`\text{When x = 5:}`

`√((5)+4) =2-(5)`

`√(9)=-3`

`3\\eq -3`
`\boxed{FALSE}`

Hope this helps!! :)

Please let me know if you have any questions