Question

Math help ASAP!! Also both drop down boxes are the same.

Answer 1

Domain: amount of fuel in the airplane's tank (in gallons)

The set of all real numbers from 0 to 200

Range: weight of airplane (In pounds)

The set of all real numbers from 3000 to 4400

Explanation:

We have the following function

`W=7F+3000`

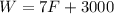
Where W represents the weight of the plane in pounds and F represents the amount of fuel in gallons.

The domain of a function is the set of values ​​"F" that can be entered in a function W(F) to obtain an output value of W.

In this case the range of the function W(F) is the whole set of values
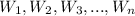`W_1, W_2, W_3, ..., W_n` that are obtained for
`F_1, F_2, F_3, ..., F_n`

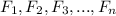
Note that, in this case, equation W(F) is used to obtain the weight of the airplane from the amount of fuel F.

Then the domain of the function is the amount of fuel in the airplane tank (in gallons). Since the tank can only hold up to 200 gallons, and there are no negative volume units, then the domain is all real numbers between 0 and 200.

The range of the function is the weight of the plane (in pounds). Note that the minimum weight of the airplane with 0 gallons of fuel is 3000 pounds and the maximum weight with the full tank is 4400 pounds.

Then the range is all real numbers between 3000 and 4400