Question

Guess a solution to y'' + y' + y = 5.

Answer 1

general solution=
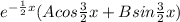`e^{-(1)/(2) x}(Acos(3)/(2) x+Bsin(3)/(2)x)`+5

Explanation:

using linear differential equation method

y'' + y' + y = 5

writing down the characteristics equation.

`m^2+m+1=0`

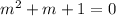
using quadratic formula

`m=(-b\pm √(b^2-4ac))/(2a)`

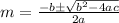
we get

`m=(-1\pm √(1^2-4(1)(1)))/(2(1))`

`m=-(1)/(2) \pm (3)/(2) i`

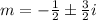
now Complementary function(CF)

`y=e^(ax)(Acosbx+Bsinbx)\\y=e^{-(1)/(2) x}(Acos(3)/(2) x+Bsin(3)/(2)x)`

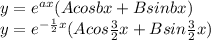
now for particular integrals

`D^2y+Dy+y=5\\(D^2+D+1)y=5\\y=(5)/(D^2+D+1)`

`P.I.=(5* e^(0x) )/(D^2+D+1)`

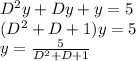
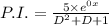
putting D=0

we get

P.I.=5

general solution=CF+PI

general solution=
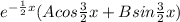`e^{-(1)/(2) x}(Acos(3)/(2) x+Bsin(3)/(2)x)`+5