Answer:

Step-by-step explanation:
The given Cauchy-Euler equation is:

Comparing to the general form:
 , we have a=1,b=-1 and c=0
, we have a=1,b=-1 and c=0
The auxiliary solution is given by:
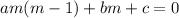


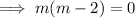
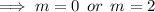
The general solution to this is of the form
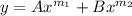 , where A and B are constants.
, where A and B are constants.

Therefore the general solution is;

Let
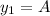 and
and
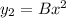
Since we CANNOT express the two solutions as constant multiple of each other, we say the two solutions are linearly independent.
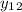 , where C is a constant.
, where C is a constant.