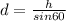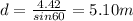Question

A 185 g block is pressed against a spring of force constant 1.60 kN/m until the block compresses the spring 10.0 cm. The spring rests at the bottom of a ramp inclined at 60.0° to the horizontal. Using energy considerations, determine how far up the incline (in m) the block moves from its initial position before it stops under the following conditions.

Answer 1

d = 5.10 m

Step-by-step explanation:

As we know that here on the plane of the inclined there is no frictional force

So in these cases we can say that total mechanical energy will always remains conserved

so here we can say that

spring potential energy = gravitational potential energy of the block

as we know from the formula

`(1)/(2)kx^2 = mgh`

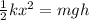
now plug in the values in it

`(1)/(2)(1.60 * 10^3)(0.10)^2 = (0.185)(9.81)h`

`8 = 1.81 h`

`h = 4.42 m`

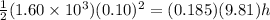
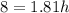
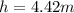
now as we know that the angle of inclination is 60 degree and height raised is 4.42 m

so here maximum distance moved along the inclined plane will be

`(h)/(d) = sin60`

`d = (h)/(sin60)`

`d = (4.42)/(sin60) = 5.10 m`