Question

A simple random sample of size nequals15 is drawn from a population that is normally distributed. The sample mean is found to be x overbarequals31.1 and the sample standard deviation is found to be sequals6.3. Determine if the population mean is different from 25 at the alpha equals 0.01 level of significance.

Answer 1

Answer with explanation:

To test the Significance of the population which is Normally Distributed we will use the following Formula Called Z test

`z=(\Bar X - \mu)/((\sigma)/(n))`

`\Bar X =31.1\\\\ \sigma=6.3\\\\ \mu=25\\\\n=15\\\\z=(31.1-25)/((6.3)/(√(15)))\\\\z=(6.1*√(15))/(6.3)\\\\z=(6.1 * 3.88)/(6.3)\\\\z=3.756`

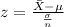
→p(Probability) Value when ,z=3.756 is equal to= 0.99992=0.9999

⇒Significance Level (α)=0.01

We will do Hypothesis testing to check whether population mean is different from 25 at the alpha equals 0.01 level of significance.

→0.9999 > 0.01

→p value > α

With a z value of 3.75, it is only 3.75% chance that ,mean will be different from 25.

So,we conclude that results are not significant.So,at 0.01 level of significance population mean will not be different from 25.