Answer:
27.The angle between two given curves is
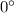 .
.
28.
Explanation:
27.We are given that two curves
y=x,y=x
We have to find the angle between the two curves
The angle between two curves is the angle between their tangent lines at the point of intersection
We know that the values of both curves at the point of intersection are equal
Let two given curves intersect at point

Then
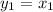 because both curves are same
because both curves are same
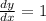
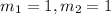
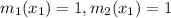
Using formula of angle between two curves

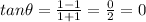
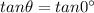
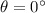
Hence,the angle between two given curves is
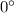 .
.
28.y=sin x
y= cos x
By similar method we solve these two curves
Let two given curves intersect at point (x,y) then the values of both curves at the point are equal
Therefore, sin x = cos x
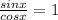
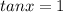
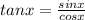
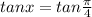
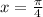
Now, substitute the value of x then we get y
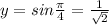
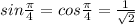
The values of both curves are same therefore, the point
 is the intersection point of two curves .
is the intersection point of two curves .

At
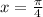
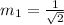
and
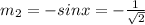
Substitute the values in the above given formula
Then we get
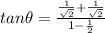
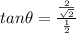
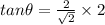


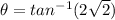
Hence, the angle between two curves is
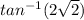 .
.