Question

1) At an axial load of 22 kN, a 15‐mm‐thick x 45‐mm‐ide polyimide polymer bar elongates 3.0 mm while the bar width contracts 0.25 mm. The bar is 200 mm long. At the 22‐kN load, the stress in the polymer bar is less than its proportional limit. Determine: a) The modulus of elasticity b) Poisson’s ratio c) The change in the bar thickness

Answer 1

Answer:2.172 GPa

0.370

0.139 mm

Step-by-step explanation:

Load[P]=22 KN

thickness[t]=15 mm

width[w]=45mm

length[L]=200 mm

Longitudnal strain
`\varepsilon _(l0)=[tex](3)/(200)`=0.015

modulus of elasticity[E]=
`(PL)/(\Delta L\ A)`

E=
`\frac{22* 10^(3)* 0.2}{15* 45* \10{-9}* 3}`

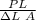
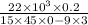
E=2.172 GPa

[b]poisson's ratio
`\mu`

`\mu =(Lateral strain)/(longitudnal strain)`

`\mu =(200* 0.25)/(3* 45)`

`\mu =0.370`

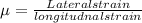
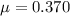
[c]Change in bar thickness

As volume remains constant

`15* 45* 200=203* 44.75* t'`

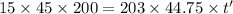
t'=14.86mm

change in thickness =0.139 mm[compression]