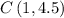Answer:
The point that splits the segment AB in half is
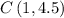
Explanation:
Given: Point A is located at
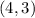 and point
and point

To find: Point that splits segment AB in half.
Solution: Let
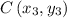 be the point that splits AB in half.
be the point that splits AB in half.
We know that the mid point
 of a line segment joining the points
of a line segment joining the points
 and
and
 is calculated as
is calculated as
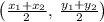
Here,
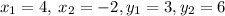
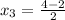
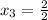
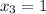
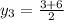
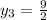
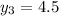
Hence, the point that splits the segment AB in half is