Answer:
At time t=0.5, the stone reaches a maximum height of h= 35.
Explanation:
A quadratic function or function of the second degree is a polynomial function defined by:
f(x)=a*x² + b*x +c
The maximums and minimums of a function are the largest (maximum) or smallest (minimum) values of a function.
The vertex of the function is a point that is part of the parabola or quadratic function that coincides with the maximum or minimum. When a> 0, the vertex of the parabola is at the bottom of it, being a minimum (that is, the parabola opens "upward"), and when a <0 the vertex is at the top , being a maximum (that is, the parable opens "downward").
h represents the value of its abscissa (value of x).
k represents the value of its ordinate (value of f (x)).
The value of h can be calculated with the formula
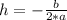
The value of k must be obtained by substituting the value of h in the function f(x)=a*x² + b*x +c
In this case: a=-16, b=16 and c=32. As a <0 the vertex is at the top , being a maximum. So:
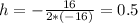
Substituting the value of h in the function f(x)=-16*x² + 16*x +32 you get:
f(0.5)=-16*0.5² + 16*0.5 +32
f(0.5)= 35
At time t=0.5, the stone reaches a maximum height of h= 35.