Question

For the first-order reaction, 2 N2O(g) → 2 N2(g) + O2(g), what is the concentration of N2O after 3 half-lives if 0.25 mol of N2O is initially placed into a 1.00-L reaction vessel?

Answer 1

Answer: The concentration of
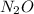`N_2O` after 3 half-lives is 0.03125 M.

Step-by-step explanation:

• To calculate the concentration of
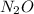  `N_2O`, we use the equation:

`\text{Molarity of the solution}=\frac{\text{Moles of solute}}{\text{Volume of solution (in L)}}`

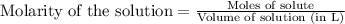
We are given:

Moles of
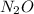`N_2O` = 0.25 moles

Volume of solution = 1 L

Putting values in above equation, we get:

`\text{Initial concentration of }N_2O=(0.25mol)/(1L)=0.25M`

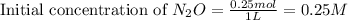
• To calculate the concentration of
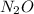  `N_2O` after given half-lives, we use the equation:

`a=(a_o)/(2^n)`

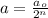
where,

a = concentration of reactant left after n-half lives = ?

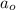`a_o` = Initial concentration of the reactant = 0.25 mol/L

n = number of half lives = 3

Putting values in above equation, we get:

`a=(0.25)/(2^3)`

`a=0.03125M`

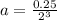
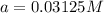
Hence, the concentration of
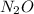`N_2O` after 3 half-lives is 0.03125 M.