Question

Aluminum has a magnetic susceptibility χ = 1.7 × 10-5 at T = 300 K. What will be the magnetization of a small sample of aluminum placed in a 1.5 T magnetic field at T = 150 K?

Answer 1

The magnetization of a small sample of aluminum is 40.6 A/m

Step-by-step explanation:

Given that,

Magnetic susceptibility
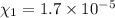`\chi_(1)=1.7*10^(-5)` at 300 K

Magnetic field = 1.5 T

We need to calculate the magnetic susceptibility at 150 K

`(\chi_(2))/(\chi_(1))=(T_(1))/(T_(2))`

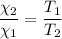
Put the value into the formula

`(\chi_(2))/(1.7*10^(-5))=(300)/(150)`

`\chi_(2)=(300*1.7*10^(-5))/(150)`

`\chi_(2)=3.4*10^(-5)`

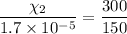
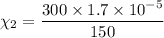
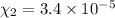
We need to calculate the magnetization

Using formula of magnetization

`I=\chi_(2)* H`

Where, H = magnetic intensity

Formula of magnetic intensity

`H=(B)/(\mu_(0))`

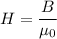
Where, B = magnetic field

Put the value of H into the formula of magnetization

`I=\chi_(2)*(B)/(\mu_(0))`

`I=3.4*10^(-5)*(1.5)/(4\pi*10^(-7))`

`I=40.6\ A/m`

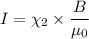
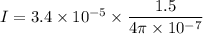
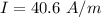
Hence, The magnetization of a small sample of aluminum is 40.6 A/m