Answer:
DNE
Explanation:
Given that two particles travel along the space curves
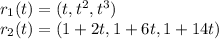
To find the points of intersection:
At points of intersection both coordinates should be equal.
i.e. r1 =r2
Equate corresponding coordinates
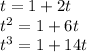
I equation gives t =-1
Substitute in II equation to get
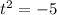
i.e. t cannot be real
Hence no point of intersection
DNE