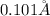Question

Technetium (Tc; Z = 43) is a synthetic element used as a radioactive tracer in medical studies. A Tc atom emits a beta particle (electron) with a kinetic energy (Ek) of 4.71 × 10−15 J. What is the de Broglie wavelength of this electron (Ek = mu2/2)?

Answer 1

Answer : The de-Broglie wavelength of this electron,
`0.101\AA`

Explanation :

The formula used for kinetic energy is,

`K.E=(1)/(2)mv^2` ..........(1)

According to de-Broglie, the expression for wavelength is,

`\lambda=(h)/(mv)`

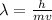
or,

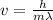`v=(h)/(m\lambda)` ...........(2)

Now put the equation (2) in equation (1), we get:

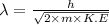`\lambda=(h)/(√(2* m* K.E))` ...........(3)

where,

`\lambda` = wavelength = ?

h = Planck's constant =
`6.626* 10^(-34)Js`

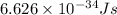
m = mass of electron =
`9.11* 10^(-31)Kg`

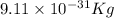
K.E = kinetic energy =
`4.71* 10^(-15)J`

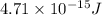
Now put all the given values in the above formula (3), we get:

`\lambda=\frac{6.626* 10^(-34)Js}{\sqrt{2* 9.11* 10^(-31)Kg* 4.71* 10^(-15)J}}`

`\lambda=1.0115* 10^(-11)m=0.101\AA`

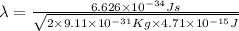
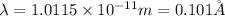
conversion used :
`(1\AA=10^(-10)m)`

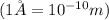
Therefore, the de-Broglie wavelength of this electron,
`0.101\AA`