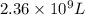Answer : The final volume of gas will be,
Explanation :
Charles' Law : It is defined as the volume of gas is directly proportional to the temperature of the gas at constant pressure and number of moles.
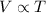
or,
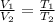
where,
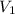 = initial volume of gas =
= initial volume of gas =

 = final volume of gas = ?
= final volume of gas = ?
 = initial temperature of gas =
= initial temperature of gas =
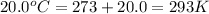
 = final temperature of gas =
= final temperature of gas =
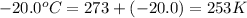
Now put all the given values in the above formula, we get the final volume of the gas.
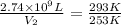
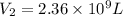
Therefore, the final volume of gas will be,