Answer: 2.14 %
Explanation:
Given : pH measurements of a chemical solutions have
Mean :
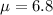
Standard deviation :

Let X be the pH reading of a randomly selected customer chemical solution.
We assume pH measurements of this solution have a nearly symmetric/bell-curve distribution (i.e. normal distribution).
The z-score for the normal distribution is given by :-

For x = 6.74
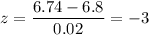
For x = 6.76
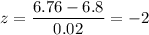
The p-value =
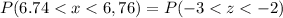
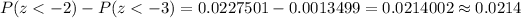
In percent,
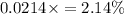
Hence, the percent of pH measurements reading below 6.74 OR above 6.76 = 2.14%