Answer:
The correct option is A.
Explanation:
If a graph is formed by connecting a single universal vertex to all vertices of a cycle, then it is known as wheel graph.
W₆ means wheel graph having 6 vertices as shown in the below figure.
Total number of edges in a wheel graph is 2(n-1), where n is number of vertices. So, the number of edges in W₆ is

In a spanning tree all the vertices covered with minimum possible number of edges. Total number of edges in a spanning tree is (n-1).
Total number of edges in a spanning tree which has 6 vertices is
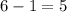
The number of edges we need to remove is
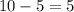
Therefore the correct option is A.