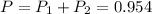Answer:
P=0.954 or 95.4%
Explanation:
Using the formula for the standardized normal distribution to find Z:

Where μ is the mean (μ=20) and σ is the standard deviation (σ=2.6).
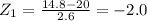
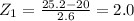
In the table of the normal distribution, we can look for positive values z, and these values are going to represent the area under the curve between z=0 and the values searched. the negatives values are found by symmetry (with the corresponding positive value but remember this area is under the left side of the curve). To find a value in the table, find the units in the first column and the follow over the same row till you find the decimals required.
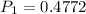

 represents the probability of length being between 14.8 and 20 (the mean) and
represents the probability of length being between 14.8 and 20 (the mean) and
 represents the probability of length being between 20 and 25.2, The requested probability is the sum of these two.
represents the probability of length being between 20 and 25.2, The requested probability is the sum of these two.