Question

Are triangles DEF and LNM similar if LN equals 4, MN equals 3, DE equals 12, and FE equals 9?

triangles LNM and DEF with angles N and E marked with the right angle symbol


Yes, by the SAS Similarity Postulate

Yes, by the SSS Similarity Postulate

No, the corresponding sides are not proportional

No, there is not enough info

Answer 1

Yes, by the SAS Similarity Postulate

Explanation:

Here, DEF and LMN are two triangles,

In which,

LN = 4 unit,

MN = 3 unit,

DE = 12 unit,

FE = 9 unit,

`(DE)/(LN)=(12)/(4)=3`

`(FE)/(MN)=(9)/(3)=3`

`\implies (DE)/(LN)=(FE)/(MN)`

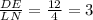
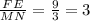
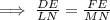
Also, m∠DEF = m∠LMN = 90° ( given )

By the SAS similarity postulate ( two corresponding sides are in same proportion and corresponding included angles are equal )

`\triangle DE F\sim \triangle LMN`

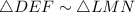
FIRST option is correct.

Answer 2

A

Explanation:

DE corrsponding to the LN, EF corrsponding to the MN, and LN:DE=MN:FE=3

and angel N is corrsponding to the angle E,so it 's SAS.