Question

47. An honest coin is tossed 10 times in a row. The result of each toss (H or T) is observed. Find the probability of the event E= “a T comes up at least once.” (Hint: Find the probability of the complementary event.)

Answer 1

0.0488%

Explanation:

Here we have the probability of different independent events, which means that none of the previous ones affect the next. For this kind of events, the probability 'P' that a series of events occur is the multiplication of the probability of each singular event.

p1: Probability that an H be obtained: 50% (is always 50% because it is independent of the previous results)

P: The probability that H be obtained all of the 10 times. This is the complementary probability to E:(a T comes up at least once).

By the first definition given

`P=0.5^(11)=0.00048828=0.0488%`

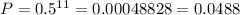
The complementary probability 'P' is the probability that 'E' does not happen, so the probability that E happen is:
`P_(E) =1-P`

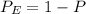
The last makes sense if we think about the fact that for the experiment there are just two possibilities, 'E' happen, or 'E' does not happen. Then,

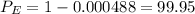`P_(E) =1-0.000488=99.95`%