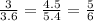Question

Which set of ratios could be used to determine if one triangle is a dilation of the other

Answer 1

See explanation

`(3.6)/(3) = (5.4)/(4.5) = (6)/(5)`

Explanation:

The two triangles are similar if the ratio of the corresponding sides are proportional.

The ratio of the corresponding sides are:

`(3.6)/(3) = 1.2`

`(5.4)/(4.5) = 1.2`

`(6)/(5) = 1.2`

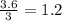
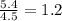
The set of ratios which could be used to determine if one triangle is a dilation of the other is

`(3.6)/(3) = (5.4)/(4.5) = (6)/(5)`

If there are multiple correct options then check this one too.

`(3)/(3.6) = (4.5)/(5.4) = (5)/(6)`