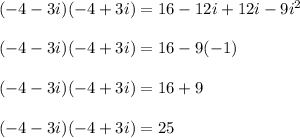Answer:
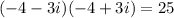
Explanation:
Notice in the graph that z1 has a real component of -4 and an imaginary component of -3.
Then we know that:
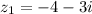
By definition for an imaginary number of the form
 its conjugate will always be the number
its conjugate will always be the number
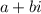
So the conjugate of
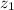 is:
is:

The product of both numbers is: