Question

Find the slope of the function f ( x ) = 2 x + 3, by the definition of limit.

Answer 1

`\displaystyle f'(x) = 2`

General Formulas and Concepts:

Calculus

Limits

Limit Rule [Constant]:
`\displaystyle \lim_(x \to c) b = b`

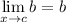
Differentiation

• Derivatives
• Derivative Notation
• Definition of a Derivative:
  `\displaystyle f'(x) = \lim_(h \to 0) (f(x + h) - f(x))/(h)`

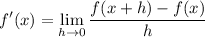
Explanation:

Step 1: Define

Identify

`\displaystyle f(x) = 2x + 3`

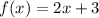
Step 2: Differentiate

1. Substitute in function [Definition of a Derivative]:
   `\displaystyle f'(x) = \lim_(h \to 0) ([2(x + h) + 3] - (2x + 3))/(h)`
2. Expand:
   `\displaystyle f'(x) = \lim_(h \to 0) (2x + 2h + 3 - 2x - 3)/(h)`
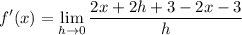
3. Combine like terms:
   `\displaystyle f'(x) = \lim_(h \to 0) (2h)/(h)`
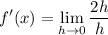
4. Simplify:
   `\displaystyle f'(x) = \lim_(h \to 0) 2`
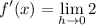
5. Evaluate limit [Limit Rule - Constant]:
   `\displaystyle f'(x) = 2`

Topic: AP Calculus AB/BC (Calculus I/I + II)

Unit: Differentiation