Question

Please help me. these problems
​

Answer 1

1st problem:

Converges to 6

2nd problem:

Converges to 504

Explanation:

You are comparing to
`\sum_(k=1)^(\infty) a_1(r)^(k-1)`

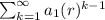
You want the ratio r to be between -1 and 1.

Both of these problem are so that means they both have a sum and the series converges to that sum.

The formula for computing a geometric series in our form is
`(a_1)/(1-r)` where
`a_1` is the first term.

The first term of your first series is 3 so your answer will be given by:

The second series has r=1/6 and a_1=420 giving me:

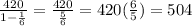`(420)/(1-(1)/(6))=(420)/((5)/(6))=420((6)/(5))=504`.