Answer:
(9,9)
Explanation:
I like to use similar triangles.
To take some confusion out of of this let's translate the line down 3 units and left 3 units.
Alright from the drawing we get:
a/8 = b/8 and a/8 = 3k/4k (or b/8=3k/4k )
We don't need the k's, they cancel.
a/8 = b/8 and a/8=3/4
So the first equation means a=b.
Now let's see what a and b are by solving a/8 = 3/4.
Cross multiply:
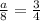
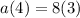
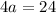
a=6
So if a=b and a=6, then b=6.
The ordered pair is (6,6).
Now let's move the line back.
We have to move it up 3 units and right 3 units which gives us the point (9,9).