Answer:
The length of AB is 352.8 feet
Explanation:
* Lets revise some facts to solve the problem
- The sine rule:
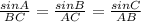
- The sum of the measures of the interior angles of a triangle is 180°
* Lets solve the problem
- In Δ ABC
∵ m∠ B = 102.9°
∵ m∠ C = 18.6°
∵ m ∠A + m∠ B + m∠ C = 180° ⇒ interior angles of a Δ
∴ m ∠A + 102.9 + 18.6 = 180
∴ m ∠A + 121.5 = 180 ⇒ subtract 121.5 from both sides
∴ m∠A = 58.5°
* Lets use the sine rule to find AB
∵ BC = 943
∵ m∠A = 58.5°
∵ m∠ C = 18.6°
∴
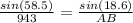
- By using cross multiplication
∴ AB × sin(58.5) = 943 × sin(18.6)
- Divide both sides by sin(58.5)
∴ AB = [943 × sin(18.6)] ÷ sin(58.5) = 352.76 ≅ 352.8
* The length of AB is 352.8 feet