Question

I want to start a lemonade stand and determine how much lemonade I need to sell to break even each day.

If I know I spend $5 on supplies and sell lemonade at $0.50 per glass determine the number of glasses I must sell to break even. After you find the number of glasses tell me what the x-intercept, y-intercept and slope. Represent in the function you determined

Answer 1

.50x -5=0
Add 5 to both sides
.50x=5
Divide both sides by.5

X =10. You must sell 10 to break even

The slope is .50
The y intercept is -5

Answer 2

Total cost spent on supplies = 5 dollars

Lemonade will be sold at 0.5 dollars.

Let the total glasses of lemonade sold be x

So, revenue generated will be = 0.5x

To get the break even we will equal both.

`0.5x=5`

`x=5/0.5`

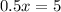
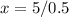
x = 10

Hence, number of glasses to be sold are 10.

The profit or y can be found as;

`y=0.5x-5`

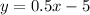
So, putting x = 11

`y=0.5(11)-5`

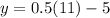
y = 0.5

Putting x = 12

`y=0.5(12)-5`

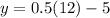
y = 1

Putting x = 13

`y=0.5(13)-5`

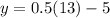
y = 1.5

The slope is 0.5.

The y-intercept is -5. This means if 0 glasses of lemonade are sold then there is a loss of $5.

The y intercept is obtained when x=0.

`y=0.5(0)-5`

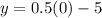
y = -5

The x-intercept is 10 or the number of glasses I must sell to break even.