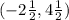Question

Square lMNO is shown in the diagram below what are the coordinates of the midpoint of a diagonal LN

Answer 1

The answer should be D the fourth one

Explanation:

Answer 2

The coordinates of the midpoint of LN are
`(-2(1)/(2),4(1)/(2))`

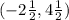
answer (4)

Explanation:

* Lets explain how to find the midpoint of a line

- The coordinates of the midpoint of a line whose endpoints are (x1 , y1)

and (x2 , y2) are
`x=(x_(1)+x_(2))/(2),y=(y_(1)+y_(2))/(2)`

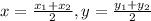
∵ LMNO is a square

∵ The coordinates of point L are (-6 , 1)

∵ The coordinates of point N are (1 , 8)

- Let the coordinates of point L are (x1 , y1) , the coordinates of point

N are (x2 , y2) and the coordinates of the midpoint of LN are (x , y)

∴ x1 = -6 , x2 = 1 and y1 = 1 , y2 = 8

- Use the rule of the midpoint above to find the midpoint (x , y)

∵
`x=(-6+1)/(2)=(-5)/(2)=-2(1)/(2)`

∵
`y=(1+8)/(2)=(9)/(4)=4(1)/(2)`

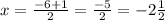
∴ The coordinates of the midpoint are
`(-2(1)/(2),4(1)/(2))`

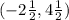
* The coordinates of the midpoint of LN are
`(-2(1)/(2),4(1)/(2))`