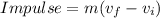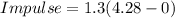Answer:
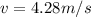

Step-by-step explanation:
Here we can say that bullet + block system is an isolated system and there is no external force on it
So Net momentum of bullet + block system will remains conserved
So we will say
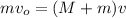
so we will have

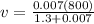
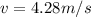
Also in order to find the impulse on the block we know that
impulse = change in the momentum of the block