Question

The length of a diagonal of a square is 24 Square root two millimeters. Find the perimeter of the square.

Select one:
o a. 576 millimeters
O b.962 millimeters
C. 96 millimeters
d. 1152 millimeters
O

Answer 1

96 mm

Explanation:

A square has 4 equal lengths. If you cut through with a diagonal, then you have form a right isosceles triangle.

So we have the diagonal, the hypotenuse, is
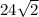`24√(2)` mm.

We need to find the side measurement of the square, one of the leg measurements of the right isosceles triangle.

Let's assume it's leg measurement is x.

So by Pythagorean Theorem we have:

`x^2+x^2=(24√(2))^2`

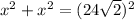
Combine like terms and simplify:

`2x^2=24^2(√(2))^2`

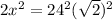
The square and square root cancel there.

`2x^2=24^2(2)`

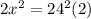
I'm going to stick 24^2 * 2 in my calculator:

`2x^2=1152`

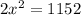
Divide both sides by 2:

`x^2=576`

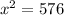
Take the square root of both sides:

`x=24`

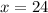
So each side of the square is 24 mm long.

To find the perimeter I add up add all the side measurements of the square or multiply 24 by 4 since all sides of square are congruent.

24(4)=96

Answer is 96 mm