Question

The angular velocity of the rear wheel of a stationary exercise bike is 5.40 rad/s at time t = 0.000 sec, and its angular acceleration is constant and equal to 1.50 rad/s^2. A particular spoke coincides with the +x axis at time t = 0.000 sec. (a) What angle (in rev) does this spoke make with the +x axis at time t = 4.00 s? (b) What is the angular velocity (rev/s) at this time?

Answer 1

The angle and angular velocity at time 4.00 are 5.35 rev and 1.81 rev/s.

Step-by-step explanation:

Given that,

Angular velocity = 5.40 rad/s

Time t = 0.000 sec

Angular acceleration = 1.50 rad/s^2

(a). We need to calculate the angle at time t = 4.00 s

Using formula for angle

`\theta=\omega_(0)t+(1)/(2)\alpha t^2`

Where,
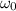`\omega_(0)`=angular velocity

`\alpha`=angular acceleration

t = time

Put the value into the formula

`\theta=5.40*4.00+(1)/(2)*1.50*(4.00)^2`

`\theta=33.6\ rad`

`\theta=(33.6)/(2\pi)\ rad`

`\theta=5.35\ rev`

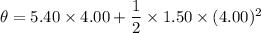
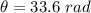
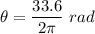
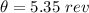
(b). We nee to calculate the angular velocity at 4.00 s

Using formula of angular velocity

`\omega=\omega_(0)+\alpha t`

`\omega =5.40+1.50*4.00`

`\omega=11.4\ rad/s`

`\omega=(11.4)/(2\pi)\ rad/s`

`\omega=1.81\ rev/s`

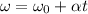
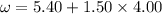
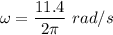
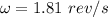
Hence, The angle and angular velocity at time 4.00 are 5.35 rev and 1.81 rev/s.