Answer with explanation:
We are asked to prove by the method of mathematical induction that:
3n(n+1) is divisible by 6 for all positive integers.
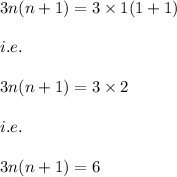
which is divisible by 6.
Hence, the result is true for n=1
- Let the result is true for n=k
i.e. 3k(k+1) is divisible by 6.
- Now we prove that the result is true for n=k+1
Let n=k+1
then
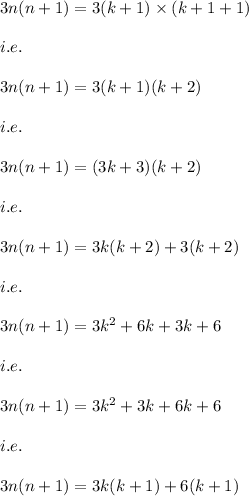
Since, the first term:
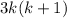 is divisible by 6.
is divisible by 6.
( As the result is true for n=k)
and the second term
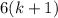 is also divisible by 6.
is also divisible by 6.
Hence, the sum:
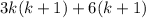 is divisible by 6.
is divisible by 6.
Hence, the result is true for n=k+1
Hence, we may say that the result is true for all n where n belongs to positive integers.