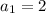Question

Enter a recursive rule for the geometric sequence.

2,−6,18,−54,...

Answer 1

see explanation

Explanation:

A recursive rule allows us to obtain any term in the sequence from the previous term.

These are the terms of a geometric sequence with common ratio r

r = - 6 ÷ 2 = 18 ÷ - 6 = - 54 ÷ 18 = - 3

Thus to obtain a term in the sequence multiply the previous term by - 3

`a_(n+1)` = - 3
`a_(n)` with a₁ = 2

Answer 2

`a_n=-3a_(n-1)` where
`a_1=2`

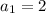
Explanation:

Recursive means you want to define a sequence in terms of other terms of your sequence.

The common ratio is what term divided by previous term equals.

The common ratio here is -6/2=18/-6=-54/18=-3.

Or in terms of the nth and previous term we could say:

`(a_n)/(a_(n-1))=r`

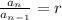
where r is -3

`(a_n)/(a_(n-1))=-3`

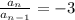
Multiply both sides by the a_(n-1).

`a_n=-3a_(n-1)` where
`a_1=2`