Question

For a 4-bar linkage with ri =7-in, r2 =3-in, r3= 9-in, and r =8-in, determi the minimum and maximum transmission angles.

Answer 1

`\mu_(min)`=
`26.38^(\circ)`

`\mu_(max)`=
`71.79^(\circ)`

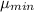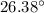
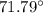
Explanation:

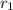`r_(1)`=7 in,
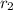`r_(2)`=3 in,
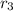`r_(3)`=9in

,
`r_(4)`=8 in

Transmission angle (μ ):

It is the acute angle between coupler and the output (follower) link.

Here we consider link
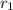`r_(1)` as fixed link ,
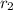`r_(2)` as input link ,link
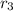`r_(3)` as coupler and link
`r_(4)` as output link.

As we know that

`\cos\mu_(max)=(r_(4)^2+r_(3)^2-r_(1)^2-r_(2)^2)/(2r_(3)r_(4))-\frac{r_(1)r_(2)}{{r_(3)r_(4)}}`

`\cos\mu_(min)=(r_(4)^2+r_(3)^2-r_(1)^2-r_(2)^2)/(2r_(3)r_(4))+\frac{r_(1)r_(2)}{{r_(3)r_(4)}}`

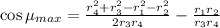
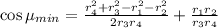
When link
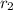`r_(2)` will be horizontal in left side direction then transmission angle will be minimum and when link
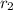`r_(2)` will be horizontal in right side direction then transmission angle will be maximum.

Now by putting the values we will find

`\cos\mu_(max)=(r_(4)^2+r_(3)^2-r_(1)^2-r_(2)^2)/(2r_(3)r_(4))-\frac{r_(1)r_(2)}{{r_(3)r_(4)}}`

`\cos\mu_(max)=0.3125`

`\mu_(max)=71.79^\circ`

`\cos\mu_(min)=(r_(4)^2+r_(3)^2-r_(1)^2-r_(2)^2)/(2r_(3)r_(4))+\frac{r_(1)r_(2)}{{r_(3)r_(4)}}`

`\cos\mu_(min)=0.8958`

`\mu_(min)=26.38^\circ`

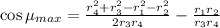
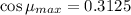
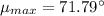
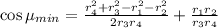
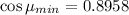
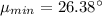
Hence, The minimum and maximum angle of transmission angle is 26.38° and 71.79° respectively.