Answer:
the rate of heat loss is 2.037152 W
Step-by-step explanation:
Given data
stainless steel K = 16 W

diameter (d1) = 10 cm
so radius (r1) = 10 /2 = 5 cm = 5 ×
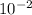
radius (r2) = 0.2 + 5 = 5.2 cm = 5.2 ×
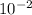
temperature = 25°C
surface heat transfer coefficient = 6 6 W
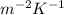
outside air temperature = 15°C
To find out
the rate of heat loss
Solution
we know current is pass in series from temperature = 25°C to 15°C
first pass through through resistance R1 i.e.
R1 = ( r2 - r1 ) / 4
 × r1 × r2 × K
× r1 × r2 × K
R1 = ( 5.2 - 5 )
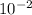 / 4
/ 4
 × 5 × 5.2 × 16 ×
× 5 × 5.2 × 16 ×
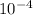
R1 = 3.825 ×

same like we calculate for resistance R2 we know i.e.
R2 = 1 / ( h × area )
here area = 4
 r2²
r2²
area = 4
 (5.2 ×
(5.2 ×
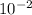 )² = 0.033979
)² = 0.033979
so R2 = 1 / ( h × area ) = 1 / ( 6 × 0.033979 )
R2 = 4.90499
now we calculate the heat flex rate by the initial and final temp and R1 and R2
i.e.
heat loss = T1 -T2 / R1 + R2
heat loss = 25 -15 / 3.825 ×
 + 4.90499
+ 4.90499
heat loss = 2.037152 W