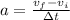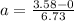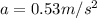Question

A girl stands on the edge of a merry-go-round of radius 1.71 m. If the merry go round uniformly accerlerates from rest to 20 rpm in 6.73s. what is the magnitiude of the girls average acceleration.

Answer 1

`a = 0.53 m/s^2`

Step-by-step explanation:

initially the merry go round is at rest

after 6.73 s the merry go round will accelerates to 20 rpm

so final angular speed is given as

`\omega = 2\pi f`

`\omega = 2\pi ( (20)/(60))`

`\omega = 2.10 rad/s`

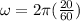
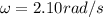
so final tangential speed is given as

`v = r\omega`

`v = 1.71 (2.10) = 3.58 m/s`

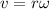
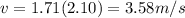
now average acceleration of the girl is given as

`a = (v_f - v_i)/(\Delta t)`

`a = (3.58 - 0)/(6.73)`

`a = 0.53 m/s^2`