Question

Raul received a score of 77 on a history test for which the class mean was 70 with a standard deviation of 6. He received a score of 79 on a biology test for which the class mean was 70 with standard deviation 4. On which test did he do better relative to the rest of the class?

Answer 1

He did better on biology test.

Explanation:

For comparing the scores we need to find z-scores for both subjects,

We know that,

z-score or standard score for x is,

`z=(x-\mu)/(\sigma)`

Where,
`\mu` is mean,

`\sigma` is standard deviation,

In history test,

`x=77`

`\mu=70`

`\sigma = 6`

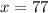
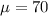
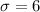
Thus, the z-score would be,

`z_1=(77-70)/(6)`

`\approx 1.167`

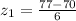
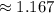
In biology test,

`x=79`

`\mu = 70`

`\sigma = 4`,

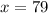
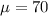
Thus, the z-score would be,

`z_2=(79-70)/(4)`

`= 2.25`

∵
`z_2>z_1`

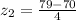
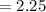
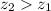
Hence, he did better on biology test.